Ms. Harley Rides to School
Ms. Harley is a teacher who saves money by riding her motorcycle to school in the morning and back home in the afternoon. It costs Ms. Harley nine cents a mile to ride her motorcycle. Ms. Harley knows the distance from her house in Greenville to Lakeland is three and forty-five hundredths miles. The distance from Lakeland to Centerville is five and forty-eight hundredths miles. The distance from Centerville to Sunrise City is one and seven hundredths miles. The distance from Sunrise City to her school is one and five tenths miles. How many total miles does Ms. Harley ride to school and back home in one day? Ms. Harley rides to school and back home five days each week for four weeks. Ms. Harley has a gas budget of fifty dollars. Does Ms. Harley stay within her budget? Show all your mathematical thinking.
Plan
Underlying Mathematical Concepts
Possible Problem-Solving Strategies
Formal Mathematical Language and Symbolic Notation
- Model
- Diagram/Key
- Table
- Number line
- Miles
- Per
- Greater than (>)/Less than (<)
- Place value (tenths, hundredths)
- Total/Sum/Product
- Distance
- Miles per hour (mph)
- Fractions (1/4, 1/2, 11 1/2)
- Decimals (3.45 ...)
- Day, week, month
- Odd/Even
- Addition
- Multiplication
- Rule (23 • d = T)
- Time
- Minutes
- Equivalent/Equal to
- Data
- Dime, dollar
- Money notation ($, .)
A student may independently select a printed number line, number chart, ten frames, graph paper, etc. as they work on a task.
Suggested Materials
Engagement Image:
Teachers may project the image below to launch this task for their students, define nouns, promote discussion, access prior knowledge, and inspire engagement and problem solving.
Possible Solutions
Assess
Novice
Novice 1
Scoring Rationale
Problem Solving
Novice
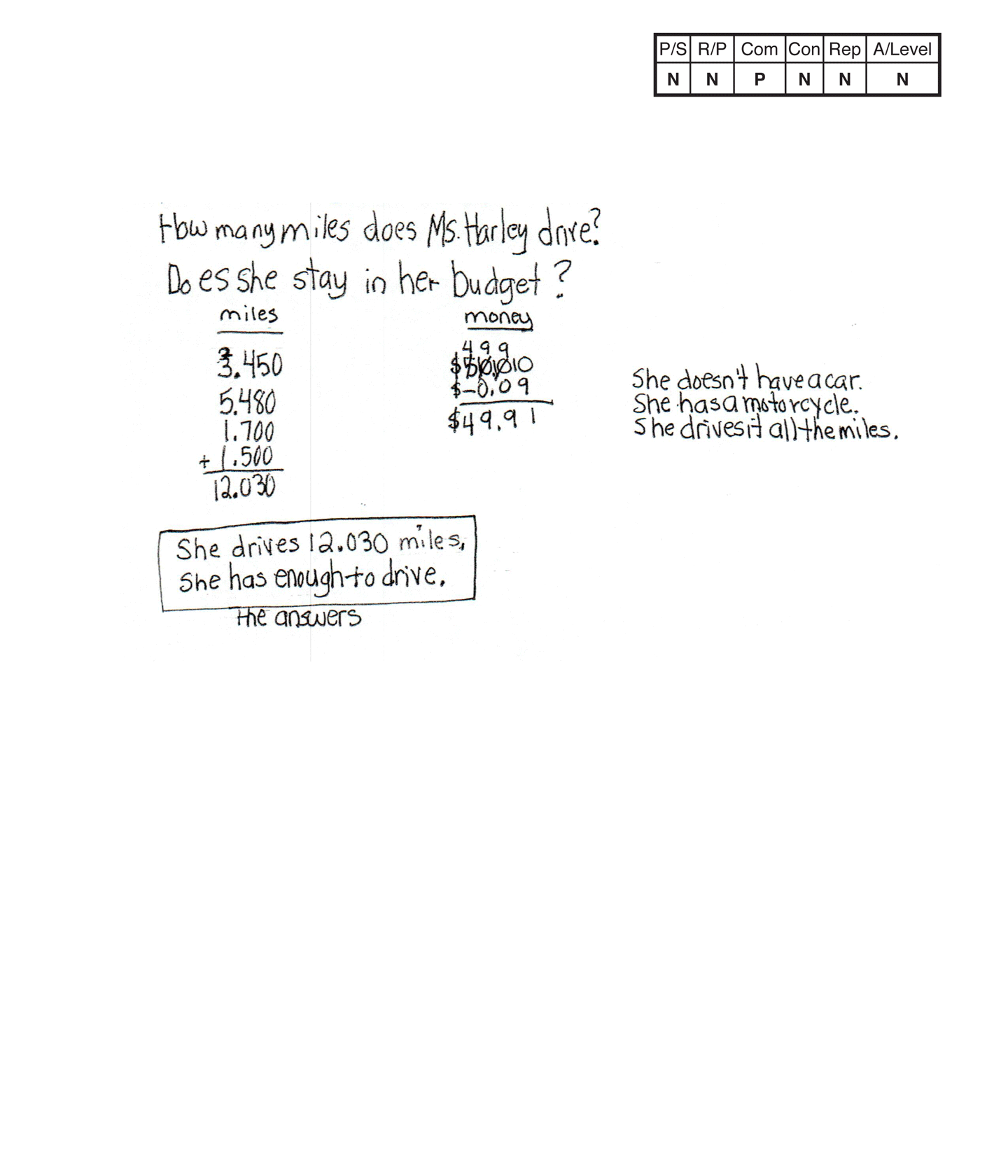
The student's strategy of listing the miles Ms. Harley rides to school in decimals to the thousandths and subtracting $0.09 from $50.00 does not work to solve the first and second parts of the task. The student's first answer, "She drives 12.030 miles," is not correct. The student's second answer, "She has enough to drive," is not correct as it is based on incorrect reasoning.
Reasoning & Proof
Novice
The student does not demonstrate correct reasoning and proof of the underlying concepts of the task. The student is not able to notate decimals to the hundreds and tenths place. It is not clear if the student's notation—1.500, represents one and five-tenths or one and five hundreds as the student lists one and seven hundredths as 1.700. The student does not understand that the miles Ms. Harley rides to school have to include total miles for a round trip. The student does not show correct reasoning that the total miles for 20 round trips has to be multiplied by $0.09 to determine if the cost does not exceed $50.00.
Communication
Practitioner
The student correctly uses the mathematical terms miles, money from the task. The student correctly notates $50.00, $0.09, $49.91. The student does not earn credit for the decimal notation as it is used incorrectly to notate either hundredths or tenths of miles.
Connections
Novice
The student's statements, "She doesn't have a car," "She has a motorcycle," and "She drives it all the miles," are not considered mathematically relevant observations.
Representation
Novice
The student does not construct a mathematical representation to support their solution.
Overall Achievement Level:
Novice
Apprentice
Apprentice 1
Scoring Rationale
Problem Solving
Apprentice
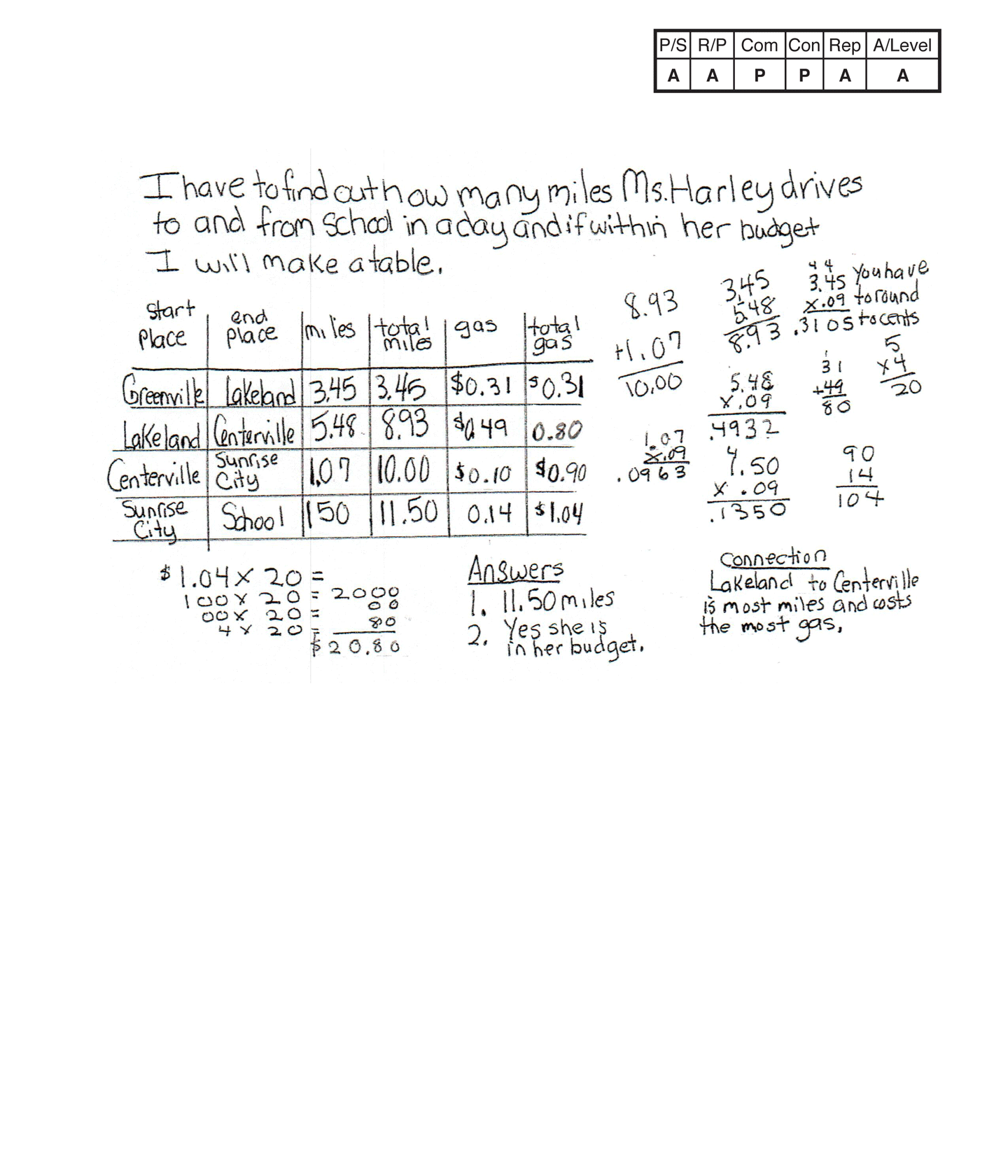
The student's strategy of using a table to show the total miles Ms. Harley rides to school and the total gas Ms. Harley uses to ride to school works to solve part of the task. The student does not consider that the task requires miles and gas used for 20 round trips. The student's first answer, "1. 11.50 miles," is incorrect. The student's second answer, "2. Yes she is in her budget," is not correct as it is not based on data for 20 round trips.
Reasoning & Proof
Apprentice
The student demonstrates correct reasoning for most of the underlying concepts of the task. The student correctly determines the four distances in miles and total miles Ms. Harley travels to school, the gas to travel four distances, and the total gas Ms. Harley uses. The student correctly determines that Ms. Harley rides her motorcycle to school for 20 days. The student does not show understanding that each trip should be considered a round trip. This results in the total miles and the total cost in gas stated by the student to represent only half of the answer.
Communication
Practitioner
The student correctly uses the mathematical terms miles, day, total, cents from the task. The student also correctly uses the terms table, most. The student correctly uses the mathematical notation 3.45, 5.48, 1.07, 8.93, 10.00, 11.50, $0.31, $0.49, $0.10, $0.14, $0.90, $1.04, $20.80.
Connections
Practitioner
The student makes the mathematically relevant observation, "Lakeland to Centerville is most miles and costs the most gas."
Representation
Apprentice
The student's table is appropriate to the task but is not accurate. The student omits a decimal point for 1.50 miles. The student also omits a dollar sign for $0.14 and $0.80.
Overall Achievement Level:
Apprentice
 ,
, 
Apprentice 2
Scoring Rationale
Problem Solving
Practitioner
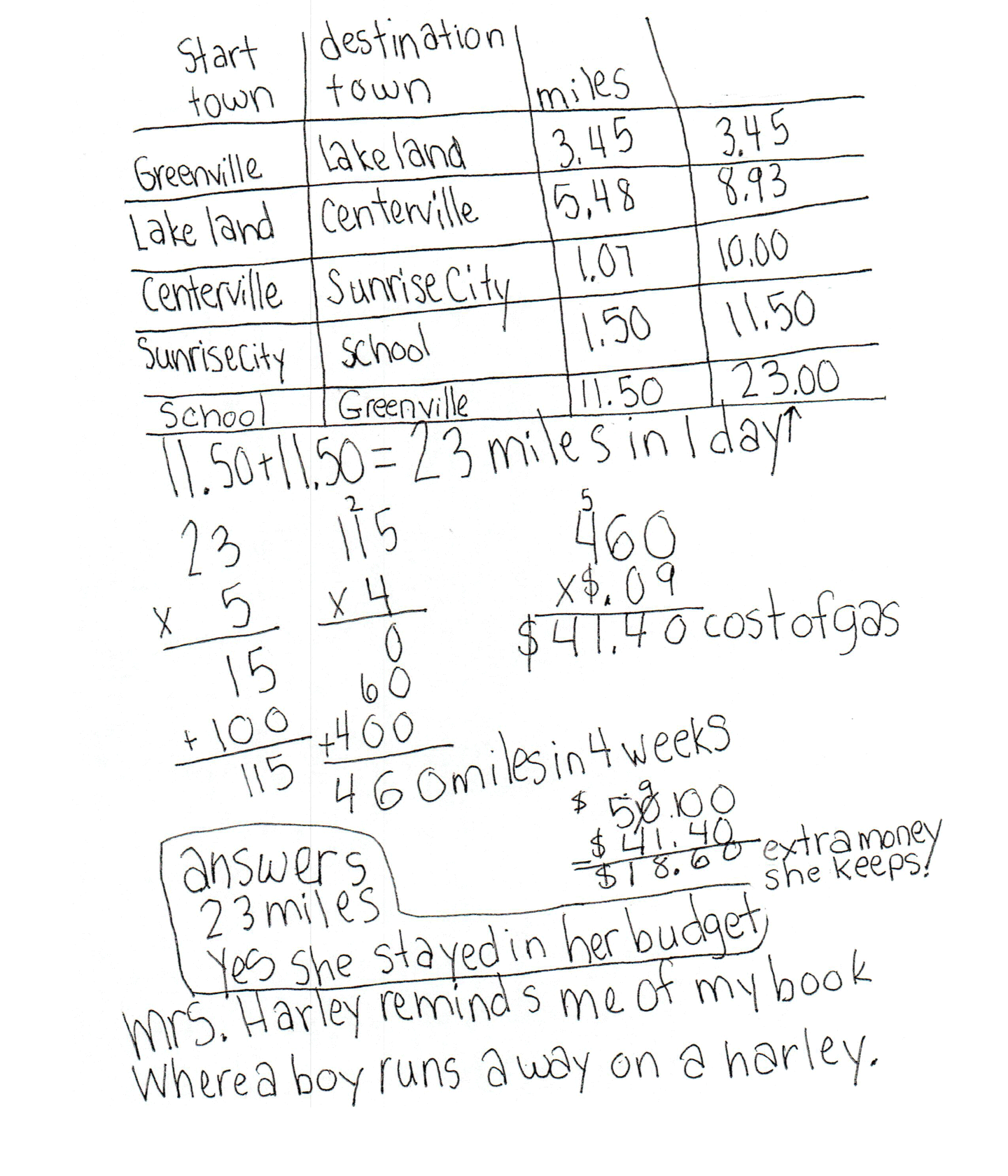
The student's strategy of using a table to show the distance in miles Ms. Harley rides between locations and the total miles she drives to and from school in one day works to solve the first part of the task. The student computes the number of miles she drives in five days, the number of miles she drives in four weeks, the total amount of money Ms. Harley uses for gas, and compares that total to $50.00 to solve the second part of the task. The student's answers, "23 miles," and "Yes she stayed in her budget," are correct.
Reasoning & Proof
Practitioner
The student demonstrates correct reasoning of the underlying concepts of the task. The student correctly uses decimal notation to determine the four distances in hundredths and tenths of a mile and the total miles Ms. Harley travels to school in one day, five days and four weeks. The student demonstrates correct reasoning by computing and correctly notating money to determine the cost of gas to travel twenty round trips to school and understanding that a comparison to $50.00 is needed.
Communication
Practitioner
The student correctly uses the mathematical terms miles, days, weeks, cost from the task. The student also correctly uses the term table. The student correctly uses the mathematical notation 3.45, 5.48, 1.07, 1.50, 11.50, 8.93, 10.00, 23.00, $.09, $41.40, $50.00, $18.60.
Connections
Apprentice
The student attempts to find the difference between $50.00 and the cost of the 20 round trips, $41.40, but the student miscalculates and arrives at an incorrect connection. The student's statement, "Mrs. Harley reminds me of my book where a boy runs away on a harley," is not considered a mathematically relevant connection.
Representation
Apprentice
The student's table is appropriate to the task but is not accurate. The student's fourth column should be labeled "total miles," "running total of miles," or "total miles rode."
Overall Achievement Level:
Apprentice
Practitioner
Practitioner 1
Scoring Rationale
Problem Solving
Practitioner
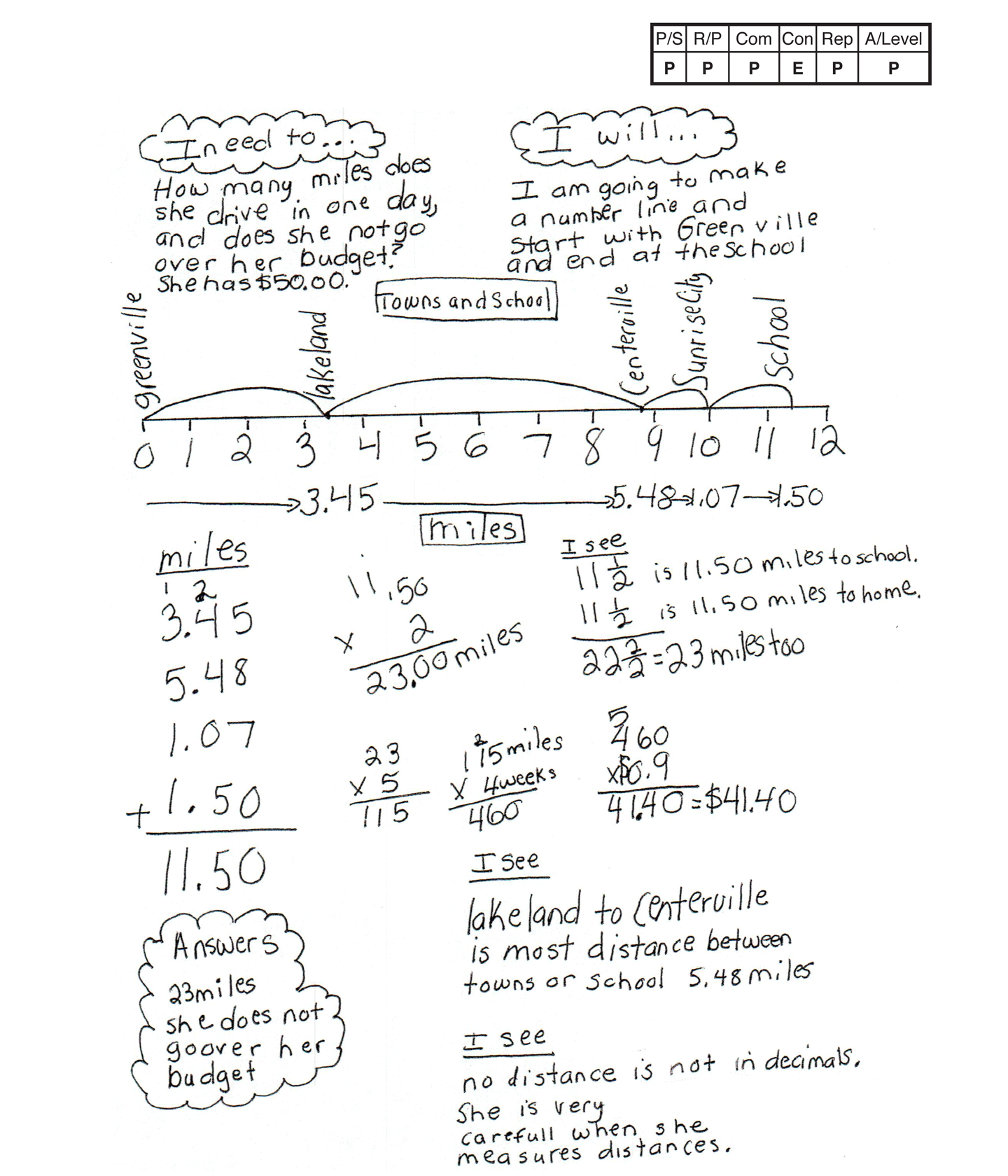
The student's strategy of using a number line to show the distance in miles Ms. Harley rides between locations from home to school works to solve the first part of the task. The student solves the rest of the task by computing the number of miles she drives in a round trip, in five days, four weeks and, how much money Ms. Harley uses for gas. The student's answers, "23 miles," and "she does not go over her budget," are correct.
Reasoning & Proof
Practitioner
The student demonstrates correct reasoning of the underlying concepts of the task. The student correctly uses decimal notation to determine the four distances in hundredths and tenths of a mile and the total miles Ms. Harley travels to school and home in one day. The student demonstrates correct reasoning by computing to find the total round trip miles for one day, five days, and four weeks. The student demonstrates correct reasoning for computing and notating money to determine the cost of gas to travel 20 round trips to school and comparing that cost to $50.00. The student also shows conceptual understanding of showing a decimal as its equivalent fraction and simplifying the fraction.
Communication
Practitioner
The student correctly uses the mathematical terms miles, day, weeks, distance, cost from the task. The student also correctly uses the terms number line, most, decimals. The student correctly uses the mathematical notation 3.45, 5.48, 1.07, $50.00, 1.50, 11.50, 23.00, $.09, $41.40, 11 1/2, 22 2/2.
Connections
Expert
The student makes mathematically relevant Practitioner connections. The student states, "Lakeland to Centerville is most distance between towns or school 5.48 miles," and "no distance is not in decimals. She is very careful when she measures distances." The student makes an Expert connection by using calculation to explain how fractions can replace decimals and arrive at the same answer of 23 miles. The student states "I see 11 1/2 is 11.50 miles to school + 11 1/2 is 11.50 miles to home = 22 2/2 = 23 miles too."
Representation
Practitioner
The student's number line is appropriate to the task and accurate. All labels are included, the intervals are accurate, and the "jumps" are correct.
Overall Achievement Level:
Practitioner
 ,
, 
Practitioner 2
Scoring Rationale
Problem Solving
Practitioner
The student's strategy of using a table to show the distance in miles Ms. Harley rides between locations from home to school and using computation to find the total miles she rides to school and home in one day works to solve the first part of the task. The student solves the second part of the task by computing the number of miles she drives in 20 days and how much money Ms. Harley uses for gas. The student's answers, "23 miles," and "She spent $41.40 in 4 weeks. She did not go over her budget," are correct.
Reasoning & Proof
Practitioner
The student demonstrates correct reasoning of the underlying concepts of the task. The student correctly uses decimal notation to define the four distances in hundreds and tenths of a mile, and the total miles Ms. Harley travels to school and home in one day and 20 days. The student demonstrates correct reasoning by computing and using money notation to determine $41.40 as the total cost of gas needed for 20 days and comparing that total to $50.00.
Communication
Practitioner
The student correctly uses the mathematical terms miles, day, weeks, total, cost from the task. The student also correctly uses the terms number line, data, mileage, month. The student correctly uses the mathematical notation 3.45, 5.48, 1.07, 1.50, 3.45, 8.93, 10.00, 11.50, 23.00, $.09, $41.40, $50.00, $8.60.
Connections
Practitioner
The student makes mathematically relevant connections. The student states, "Her longest route part was Lake Land to centerville," "Her shortest route part was Centerville to Sunrise City." The student uses computation to determine "7 days x 4 weeks is 28 days—a month, 5 days x 4 weeks is 20 days she rides to school = 8 days at home." The student computes $50.00 - $41.40 = $8.60 and divides that difference by 9 (cents) to determine that, "she has enuf money for 95 more miles."
Representation
Practitioner
The student's table is appropriate to the task and accurate. All labels are included and all entered data is correct.
Overall Achievement Level:
Practitioner
Expert
 ,
, 
Expert 1
Scoring Rationale
Problem Solving
Expert
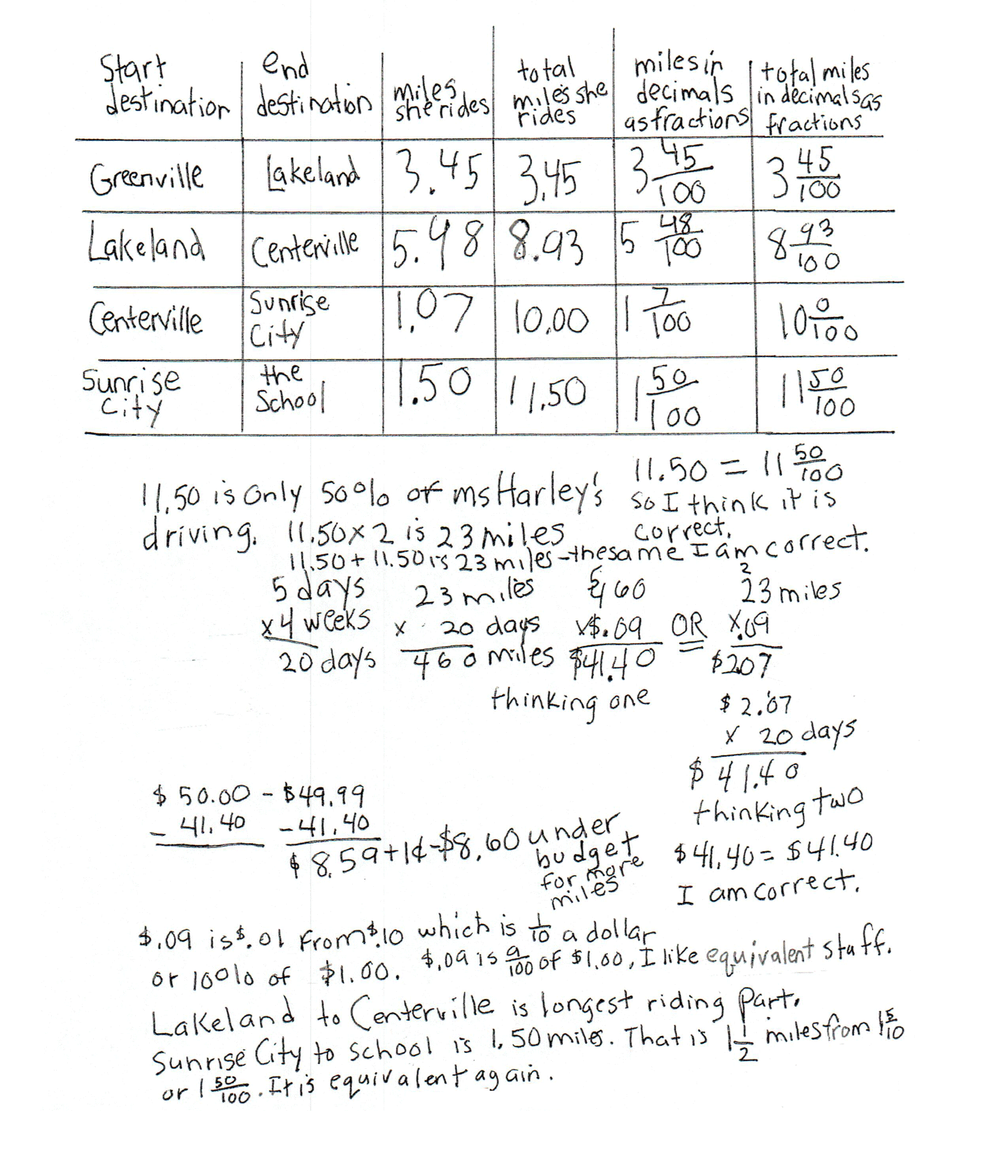
The student's strategy of using a table to show the distance in miles Ms. Harley rides between locations from home to school and using computation to find the total miles she rides to school and home works to solve the first part of the task. The student solves the rest of the task by computing the number of miles she drives in 20 days, and how much money Ms. Harley uses for gas. The student's "Conclusions, she rides 23 miles per day. She spent $41.40 per week. She did not go over budget." are correct. The student also includes fractions and percent in their solution and verifies their answers using different computation and combinations.
Reasoning & Proof
Expert
The student demonstrates correct reasoning of the underlying concepts of the task. The student correctly uses decimal notation to define the four distances in hundredths and tenths of a mile, and the total miles Ms. Harley travels to school and home in one day and 20 days. The student demonstrates correct reasoning by computing and using money notation to determine $41.40 as the total cost of gas needed for 20 days and comparing that total to $50.00. The student also demonstrates understanding of the concepts of equivalent fractions for decimals and percents. The student also justifies their answers.
Communication
Expert
The student correctly uses the mathematical terms miles, day, week, total, cost from the task. The student also correctly uses the terms table, per, decimals, fractions, dollar, equivalent, more. The student correctly uses the mathematical notation 3.45, 5.48, 1.07, 1.50, 3.45, 8.93, 10.00, 11.50, 3 45/100, 5 48/100, 1 7/100, 1 50/100, 8 93/100, 10 0/100, 11 50/100, 1/10, 9/100, 1 1/2, 1 5/10, $.09, $2.07, $41.40, $50.00, $8.59, $8.60, $.01, $.10, $1.00, 50%, 10%.
Connections
Expert
The student makes mathematically relevant Practitioner connections. The student states, "Lakeland to Centerville is longest riding part," and "$8.60 under budget for more miles." The student makes Expert connections. The student includes the equivalent mixed numbers for "miles in decimals as fractions" and "total miles in decimals as fractions" on their table. The student justifies their decisions. The student states, "11.50 if only 50% of ms Harley's driving. 11.50 x 2 is 23 miles," "11.50 + 11.50 is 23 miles—the same, I am correct," "11.50 = 11 50/100 so I think it is correct." The students uses two different strategies for computing $41.40 calling them, "thinking one" and "thinking two" and stating, "$41.40 = $41.40 I am correct." The student also supports their understanding that, "$.09 is $.01 from $.10 which is 1/10 of a dollar or 10% of $1.00," and "$.09 is 9/100 of $1.00. I like equivalent stuff." The student ends their solution by stating, "Sunrise City to school is 1.50 miles. That is 1 1/2 miles from 1 5/10 or 1 50/100. It is equivalent again."
Representation
Expert
The student's table is appropriate to the task and accurate. All labels are included and all extended data is correct. The student extends their thinking to include mixed numbers in the table and to compare 11.50 from the table to 50% and 1.50 from the table to 1 1/2 and 1 5/10 in their solution.
Overall Achievement Level:
Expert
 ,
,  ,
, 
Expert 2
Scoring Rationale
Problem Solving
Expert
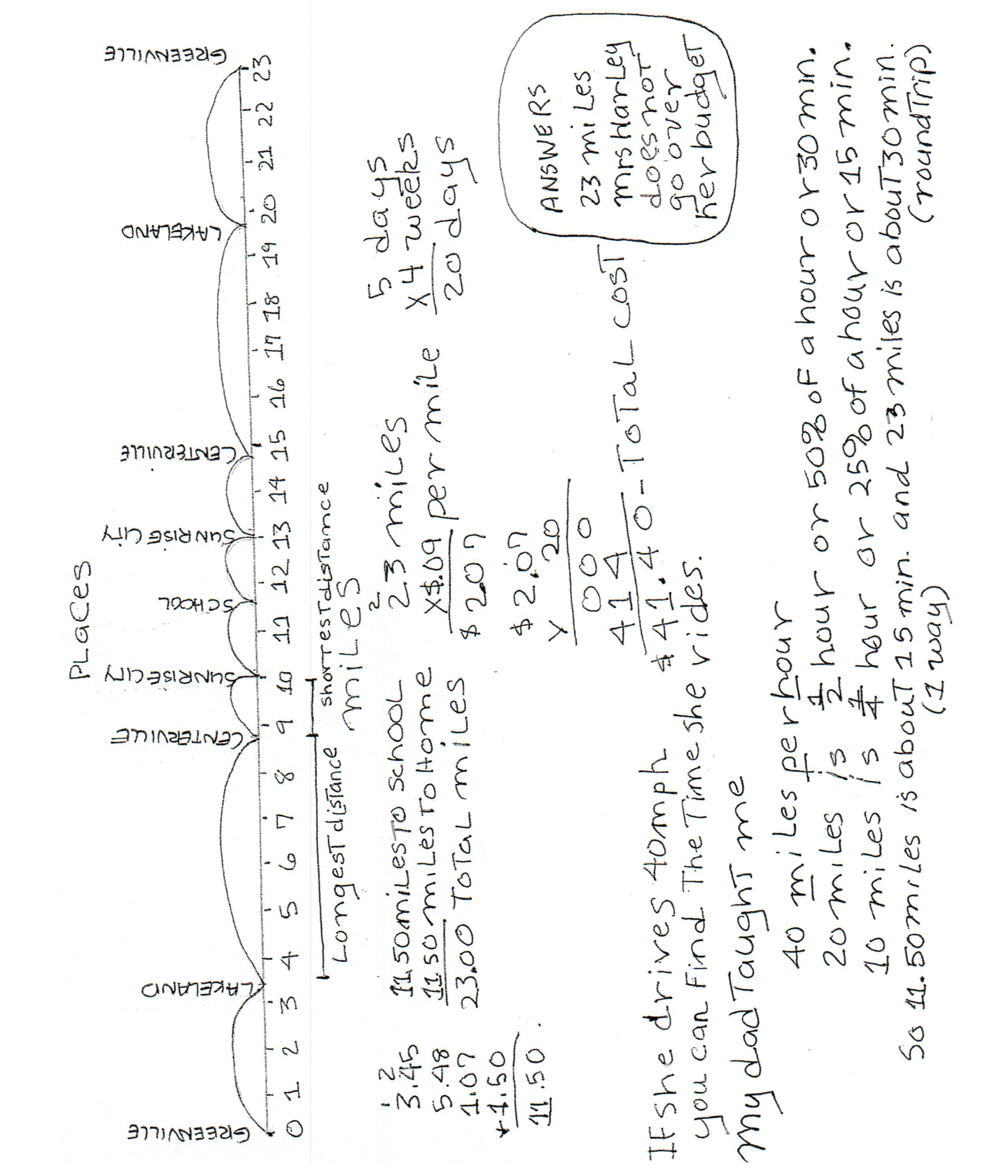
The student's strategy of using a number line and calculation to show the total distance in miles Ms. Harley rides between locations from home to school and home, works to solve the first part of the task. The student's strategy of calculating the cost of gas to ride 23 miles and the cost for riding 20 days, works to solve the second part of the task.The student's answers, "3 miles," and "Mrs Harley does not go over her budget," are correct. The student continues their solution to include percents and time measurement.
Reasoning & Proof
Expert
The student demonstrates correct reasoning of the underlying concepts of the task. The student correctly uses decimal notation to define the four distances in hundredths and tenths of a mile, and the total miles Ms. Harley travels to school and home in one day and 20 days. The student demonstrates correct reasoning by computing and using money notation to determine $41.40 as the total cost of gas needed for 20 days and comparing that total to $50.00. The student also demonstrates understanding of the concepts of fractions, percents and time measurement. The student justifies their understanding of comparing time to miles per hour and decimals and reaches a conclusion.
Communication
Expert
The student correctly uses the mathematical terms miles, distance, total, days, weeks, cost from the task. The student also correctly uses the terms number line time, per, miles per hour/mph, time, hour, min., more, decimals, fraction. The student correctly uses the mathematical notation 3.45, 5.48, 1.07, 1.50, 11.50, 23.00, .575, .5, 1.0, $.09, $2.07, $41.40, $50.00, 1/2, 1/4, 50%, 25%. <.
Connections
Expert
The student solves the task and makes the Expert connection of extending their thinking to miles per hour and how this concept relates to the task. The student states, "If she drives 40 mph you can find the time she rides." The student defines 40 mph and determines "20 miles is 1/2 hour or 50% of a hour or 30 min.," "10 miles is 1/4 hour or 25% of a hour or 15 min.," "So 11.50 miles is about 15 min. (1 way) and 23 miles is about 30 min. (round trip)." The student divides 23.00 miles by 40 miles per hour for a quotient of .575 which the student rounds to 6. The student states, "I think the 6 means 1/2 hour on a clock," The student diagrams a clock and states, "But .545 < 6 and 20 miles is 1/2 40 mph so 30 mins, 20 < 23 so it takes a little more time to ride like 34 min." The student continues their explanation. "I know .575 is like decimals. .5 + .5 = 1.0 so .575 is a little more like 23 miles is a little more. So .5 is 1/2 hour about a round trip. So .25 is 1/4 hour about 1 way." The student aligns decimals and fractions to percents. ".5 + .5 is 1 hour. 1/2 + 1/2 is 1 hour. 50% + 50% is 1 hour." The student concludes, "my clock idea was wrong becaus I was thinking the number 6 on a clock and not mph fractions!!!"
Representation
Expert
The student's table is appropriate to the task and accurate. All labels are included and all entered data is correct. The student extends their thinking to include mixed numbers in the table and to compare 11.50 from the table to 50% of 23.00, and 1.50 from the table to 1 1/2 and 1 5/10 in their solution. The student also diagrams a clock to indicate 1/2 an hour representing 30 minutes.



MP.1
Make sense of problems and persevere in solving them.