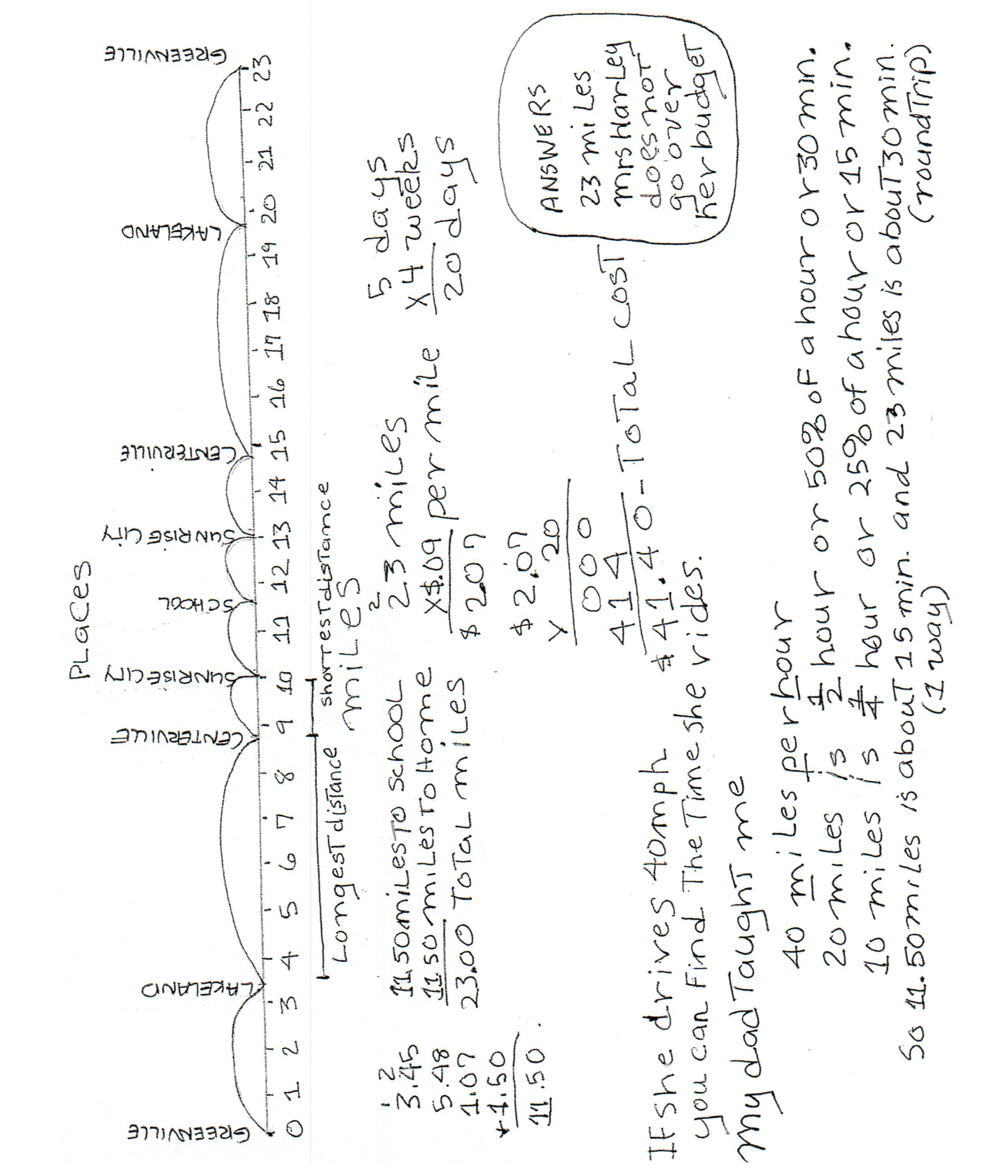
Ms. Harley Rides to School

Overall Achievement Level:

expert

Student Number:

Anchor Papers:

Problem Solving

expert

Problem Solving Rationale

The student's strategy of using a number line and calculation to show the total distance in miles Ms. Harley rides between locations from home to school and home, works to solve the first part of the task. The student's strategy of calculating the cost of gas to ride 23 miles and the cost for riding 20 days, works to solve the second part of the task.The student's answers, "3 miles," and "Mrs Harley does not go over her budget," are correct. The student continues their solution to include percents and time measurement.

Reasoning and Proof

expert

Reasoning Proof Rationale

The student demonstrates correct reasoning of the underlying concepts of the task. The student correctly uses decimal notation to define the four distances in hundredths and tenths of a mile, and the total miles Ms. Harley travels to school and home in one day and 20 days. The student demonstrates correct reasoning by computing and using money notation to determine $41.40 as the total cost of gas needed for 20 days and comparing that total to $50.00. The student also demonstrates understanding of the concepts of fractions, percents and time measurement. The student justifies their understanding of comparing time to miles per hour and decimals and reaches a conclusion.

Communication Level

expert

Communication Rationale

The student correctly uses the mathematical terms miles, distance, total, days, weeks, cost from the task. The student also correctly uses the terms number line time, per, miles per hour/mph, time, hour, min., more, decimals, fraction. The student correctly uses the mathematical notation 3.45, 5.48, 1.07, 1.50, 11.50, 23.00, .575, .5, 1.0, $.09, $2.07, $41.40, $50.00, 1/2, 1/4, 50%, 25%. <.

Connections Level

expert

Connections RationaleMs. Harley Rides to School

The student solves the task and makes the Expert connection of extending their thinking to miles per hour and how this concept relates to the task. The student states, "If she drives 40 mph you can find the time she rides." The student defines 40 mph and determines "20 miles is 1/2 hour or 50% of a hour or 30 min.," "10 miles is 1/4 hour or 25% of a hour or 15 min.," "So 11.50 miles is about 15 min. (1 way) and 23 miles is about 30 min. (round trip)." The student divides 23.00 miles by 40 miles per hour for a quotient of .575 which the student rounds to 6. The student states, "I think the 6 means 1/2 hour on a clock," The student diagrams a clock and states, "But .545 < 6 and 20 miles is 1/2 40 mph so 30 mins, 20 < 23 so it takes a little more time to ride like 34 min." The student continues their explanation. "I know .575 is like decimals. .5 + .5 = 1.0 so .575 is a little more like 23 miles is a little more. So .5 is 1/2 hour about a round trip. So .25 is 1/4 hour about 1 way." The student aligns decimals and fractions to percents. ".5 + .5 is 1 hour. 1/2 + 1/2 is 1 hour. 50% + 50% is 1 hour." The student concludes, "my clock idea was wrong becaus I was thinking the number 6 on a clock and not mph fractions!!!"

Representation

expert

Representation Rationale

The student's table is appropriate to the task and accurate. All labels are included and all entered data is correct. The student extends their thinking to include mixed numbers in the table and to compare 11.50 from the table to 50% of 23.00, and 1.50 from the table to 1 1/2 and 1 5/10 in their solution. The student also diagrams a clock to indicate 1/2 an hour representing 30 minutes.