Ms. Harley Rides to School

Overall Achievement Level:

expert

Student Number:

Anchor Papers:

Problem Solving

expert

Problem Solving Rationale

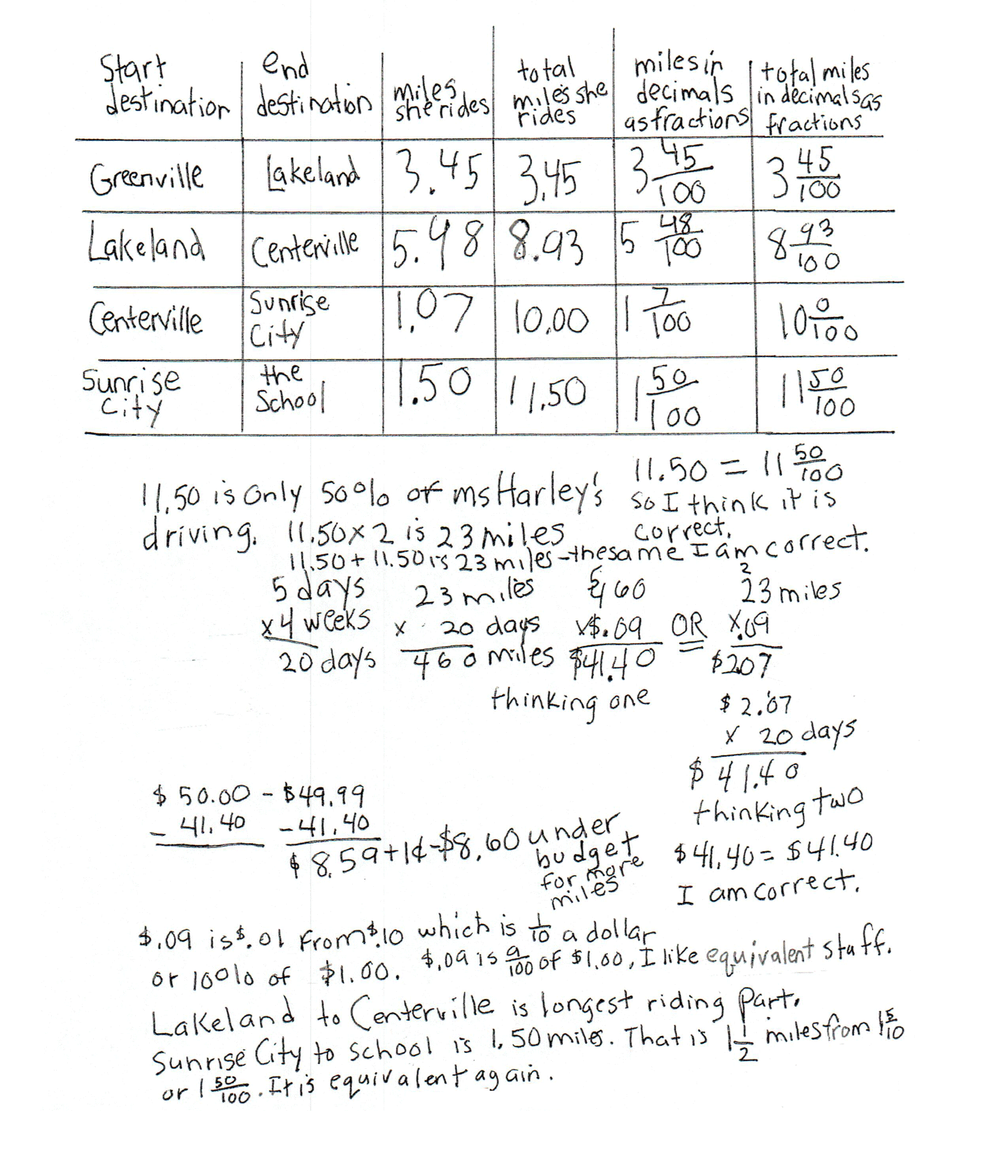
The student's strategy of using a table to show the distance in miles Ms. Harley rides between locations from home to school and using computation to find the total miles she rides to school and home works to solve the first part of the task. The student solves the rest of the task by computing the number of miles she drives in 20 days, and how much money Ms. Harley uses for gas. The student's "Conclusions, she rides 23 miles per day. She spent $41.40 per week. She did not go over budget." are correct. The student also includes fractions and percent in their solution and verifies their answers using different computation and combinations.

Reasoning and Proof

expert

Reasoning Proof Rationale

The student demonstrates correct reasoning of the underlying concepts of the task. The student correctly uses decimal notation to define the four distances in hundredths and tenths of a mile, and the total miles Ms. Harley travels to school and home in one day and 20 days. The student demonstrates correct reasoning by computing and using money notation to determine $41.40 as the total cost of gas needed for 20 days and comparing that total to $50.00. The student also demonstrates understanding of the concepts of equivalent fractions for decimals and percents. The student also justifies their answers.

Communication Level

expert

Communication Rationale

The student correctly uses the mathematical terms miles, day, week, total, cost from the task. The student also correctly uses the terms table, per, decimals, fractions, dollar, equivalent, more. The student correctly uses the mathematical notation 3.45, 5.48, 1.07, 1.50, 3.45, 8.93, 10.00, 11.50, 3 45/100, 5 48/100, 1 7/100, 1 50/100, 8 93/100, 10 0/100, 11 50/100, 1/10, 9/100, 1 1/2, 1 5/10, $.09, $2.07, $41.40, $50.00, $8.59, $8.60, $.01, $.10, $1.00, 50%, 10%.

Connections Level

expert

Connections RationaleMs. Harley Rides to School

The student makes mathematically relevant Practitioner connections. The student states, "Lakeland to Centerville is longest riding part," and "$8.60 under budget for more miles." The student makes Expert connections. The student includes the equivalent mixed numbers for "miles in decimals as fractions" and "total miles in decimals as fractions" on their table. The student justifies their decisions. The student states, "11.50 if only 50% of ms Harley's driving. 11.50 x 2 is 23 miles," "11.50 + 11.50 is 23 miles—the same, I am correct," "11.50 = 11 50/100 so I think it is correct." The students uses two different strategies for computing $41.40 calling them, "thinking one" and "thinking two" and stating, "$41.40 = $41.40 I am correct." The student also supports their understanding that, "$.09 is $.01 from $.10 which is 1/10 of a dollar or 10% of $1.00," and "$.09 is 9/100 of $1.00. I like equivalent stuff." The student ends their solution by stating, "Sunrise City to school is 1.50 miles. That is 1 1/2 miles from 1 5/10 or 1 50/100. It is equivalent again."

Representation

expert

Representation Rationale

The student's table is appropriate to the task and accurate. All labels are included and all extended data is correct. The student extends their thinking to include mixed numbers in the table and to compare 11.50 from the table to 50% and 1.50 from the table to 1 1/2 and 1 5/10 in their solution.